
|
 |
 |
 |
 |
 |
 |
(right) strophoid |
cubic |
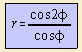
The strophoid 1) is an extension of the cissoid 2), and in
Cartesian coordinates it is written as:
![]()
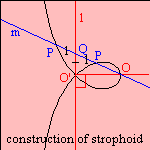
To construct the curve: given a line l, and a point O (called the pole), that is
perpendicular projected on l in O'. Make now a line bundle m through O. The strophoid is
now the collection of the points P on m for which O'Q = PQ. Q is the intersection
of l and m.
This construction is closely related to the one of the cissoid,
and it can also be seen as a specimen of the general
cissoid.
 Roberval found the curve as the result of planes cutting a cone: when the plane
rotates (about the tangent at its vertex) the collection of foci of the obtained conics
gives the strophoid.
Roberval found the curve as the result of planes cutting a cone: when the plane
rotates (about the tangent at its vertex) the collection of foci of the obtained conics
gives the strophoid.
The strophoid has the following qualities:
- the polar inverse of the strophoid
is the curve itself (with the pole as center of inversion). On the other hand, when the
node is taken as center of inversion, its inverse is the hyperbola.
- the curve is a pedal of the parabola.
- the strophoid is formed by the points of contact of parallel tangents to the
cochleoid.
- the curve is a nodal curve
In the 19th century J.Booth wrote about the curve in the Proceedings of the Royal Society of Londen (vol.9, 1857-1859). He called the curve the logocyclic curve.
The curve is also named the foliate.
The strophoid is called the right strophoid to distinguish from the more general oblique strophoid.
A strophoid plane is used as a model of beta-barrels in proteins.
1) Strophè (Gr.) = turn, swing
In Dutch: striklijn.
2) As the strophoid's equation can be rewritten as: r = cosφ - sinφ tanφ,
which is equivalent with the term cosφ added to the cissoid's equation.